Syllogism worked examples
Example 1:

1. All Makaras are Patalans.
2. Some Makaras are Svargans.
Conclusion: Some Svargans are Patalans.
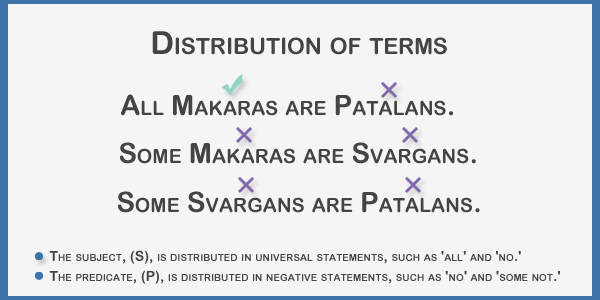
Conclusion is valid
Similar to case 7.
Rule 1: When both premises are positive, then the conclusion must be positive.
Rule 3: The middle term, B, is distributed in the major premise.
Rule 9: If a statement begins with 'some', the conclusion must begin with 'some'.
Example 2:
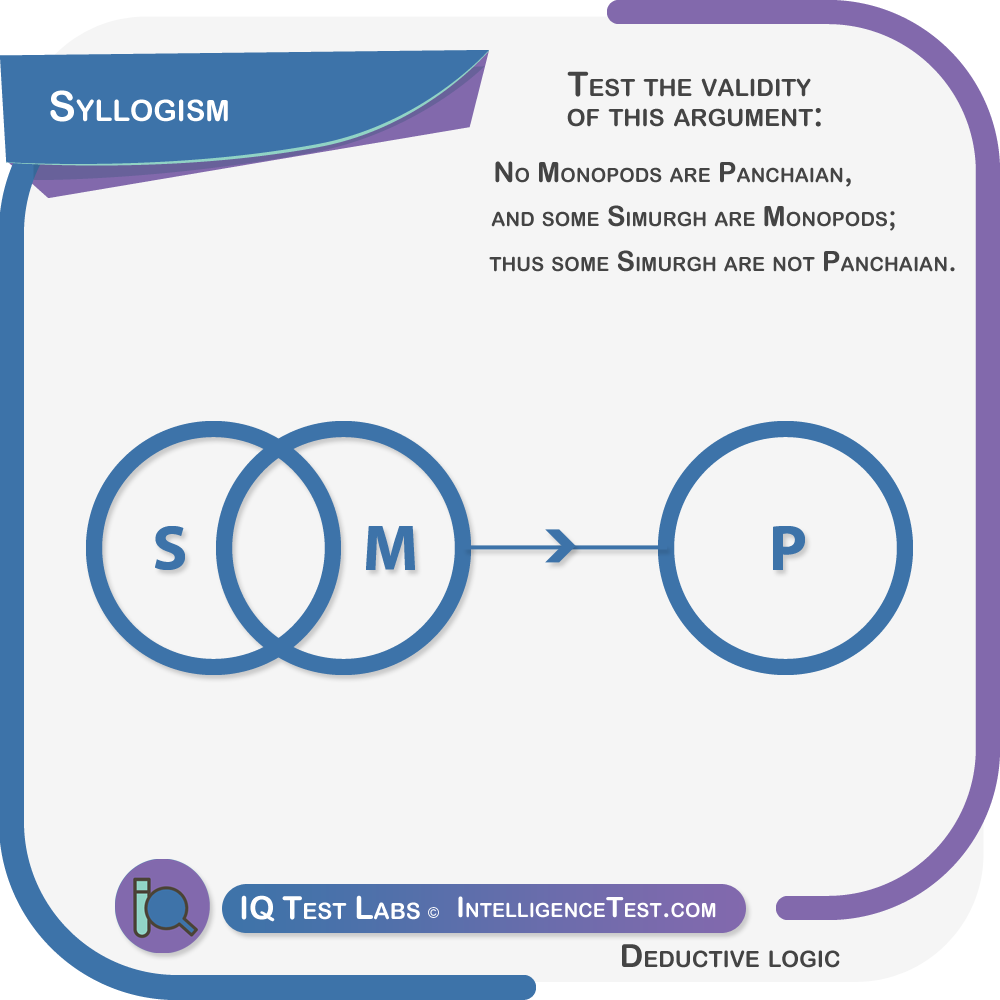
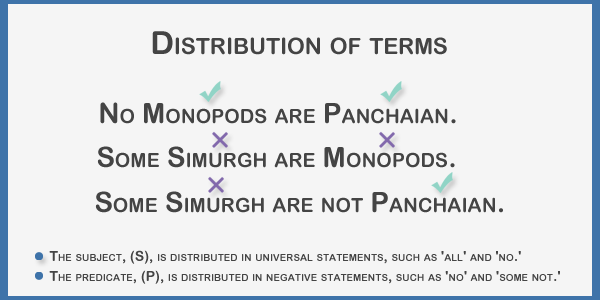
1. No Monopods are Panchaian.
2. Some Simurgh are Monopods.
Conclusion: Some Simurgh are not Panchaian.
Conclusion is valid
Similar to case 12.
Rule 2: When 'No' appears in a statement, 'Some-not' should follow as a valid possible conclusion.
Rule 3: The middle term, B, is distributed in the major premise.
Rule 4: The major term, P, is distributed in the conclusion and the major premise.
Rule 7: If one statement is negative, the conclusion must be negative.
Rule 9: If a statement begins with 'some', the conclusion must begin with 'some'.
Example 3:
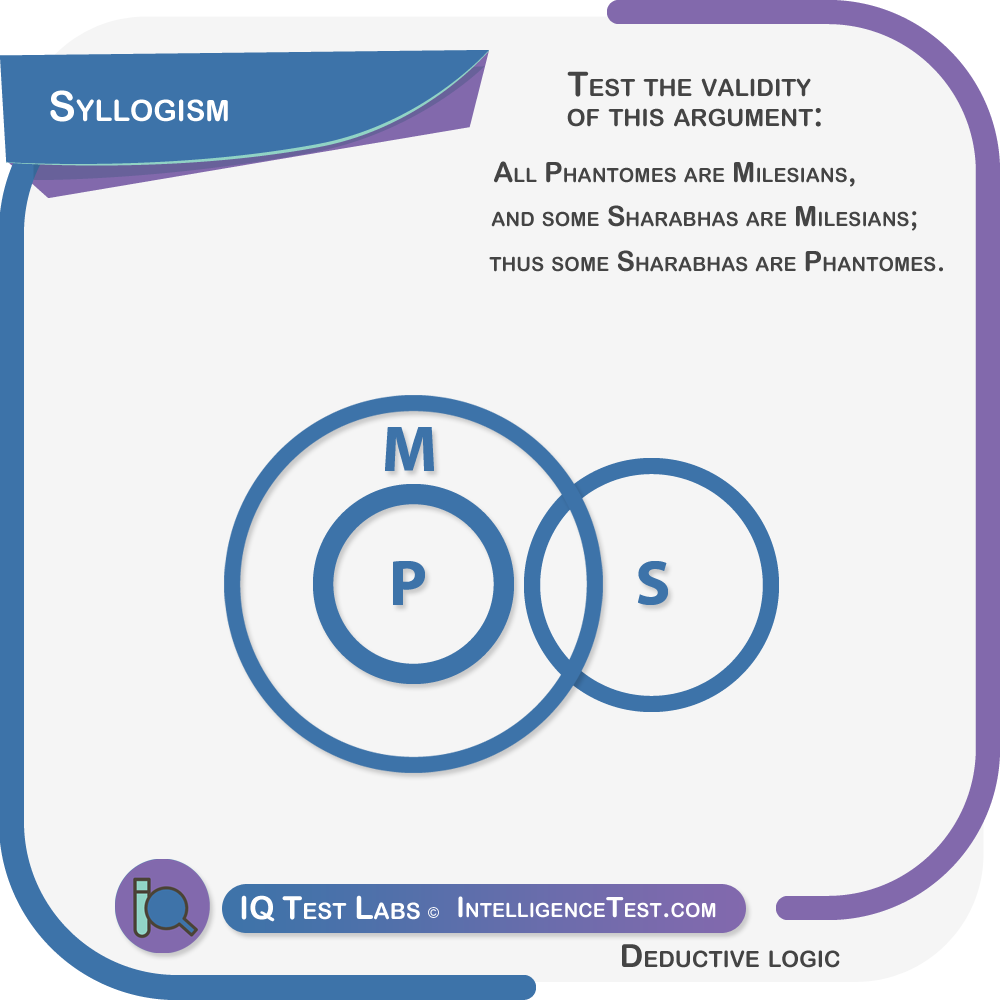
1. All Phantomes are Milesians.
2. Some Sharabhas are Milesians.
Conclusion: Some Sharabhas are Phantomes.

Conclusion is not valid
Similar to case 8.
Rule 3: The middle term, B, is not distributed in the premises.
Example 4:

1. Some Pixiu are Mag Mellians.
2. No Scyllas are Mag Mellians.
Conclusion: Some Pixiu are not Scyllas.
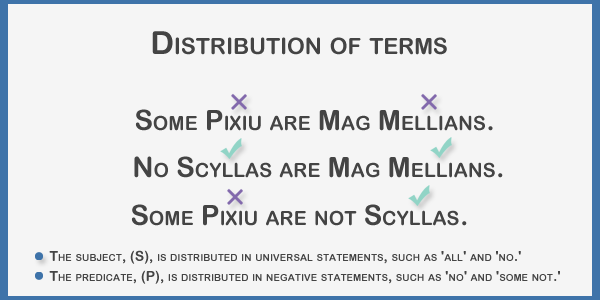
Conclusion is valid
Similar to case 13.
Rule 2: When 'No' appears in a statement, 'Some-not' should follow as a valid possible conclusion.
Rule 3: The middle term, B, is distributed in the major premise.
Rule 5: The minor term, S, is distributed in the conclusion and the minor premise.
Rule 7: If one statement is negative, the conclusion must be negative.
Rule 9: If a statement begins with 'some', the conclusion must begin with 'some'.
Example 5:

1. All Pukwudgies are Mus.
2. Some Sharbhas are not Mus.
Conclusion: Some Pukwudgies are not Sharabhas.

Conclusion is not valid
Similar to case 17.
Rule 5: Whereas the minor term, S, is distributed in the conclusion, it is not distributed in the minor premise.
Example 6:
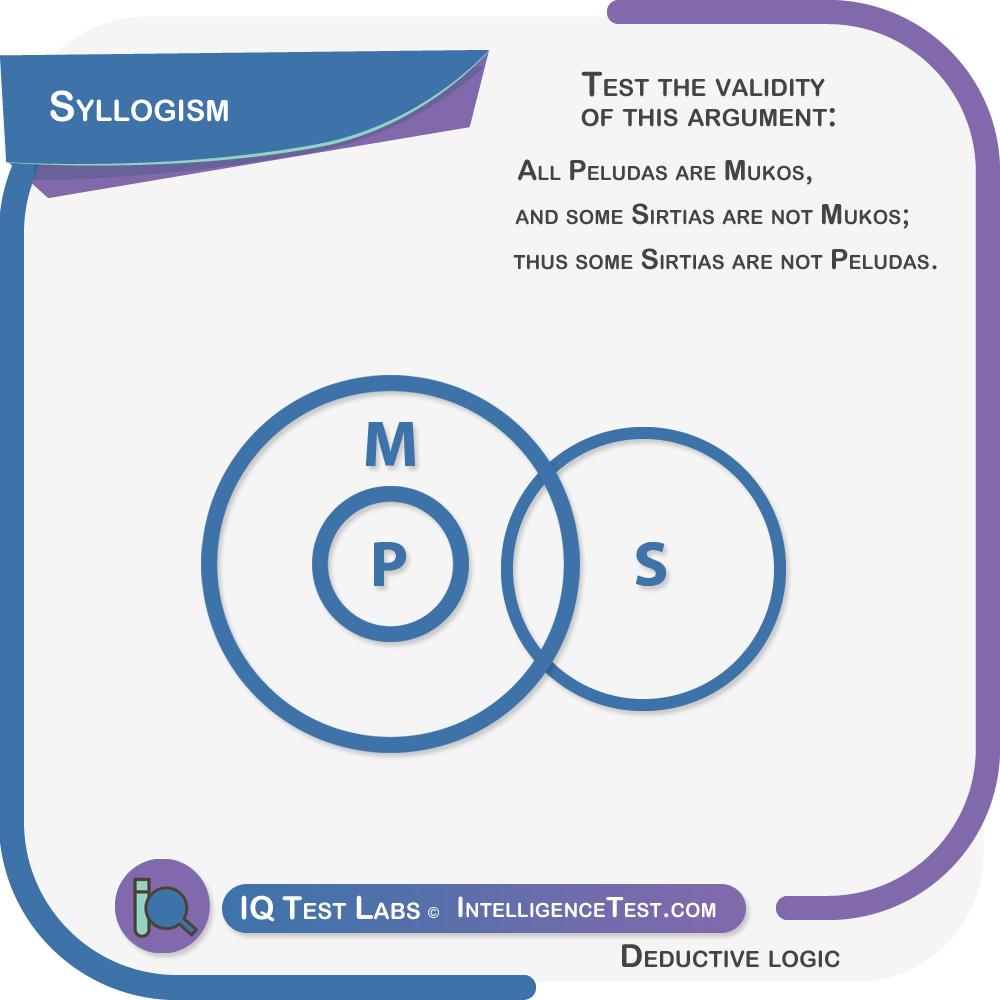
1. All Peludas are Mukos.
2. Some Sirtias are not Mukos.
Conclusion: Some Sirtias are not Peludas.

Conclusion is valid
Similar to case 17.
Rule 3: The middle term, B, is distributed in the minor premise.
Rule 4: The major term, P, is distributed in the conclusion and the major premise.
Rule 7: If one statement is negative, the conclusion must be negative.
Rule 9: If a statement begins with 'some', the conclusion must begin with 'some'.
Example 7:
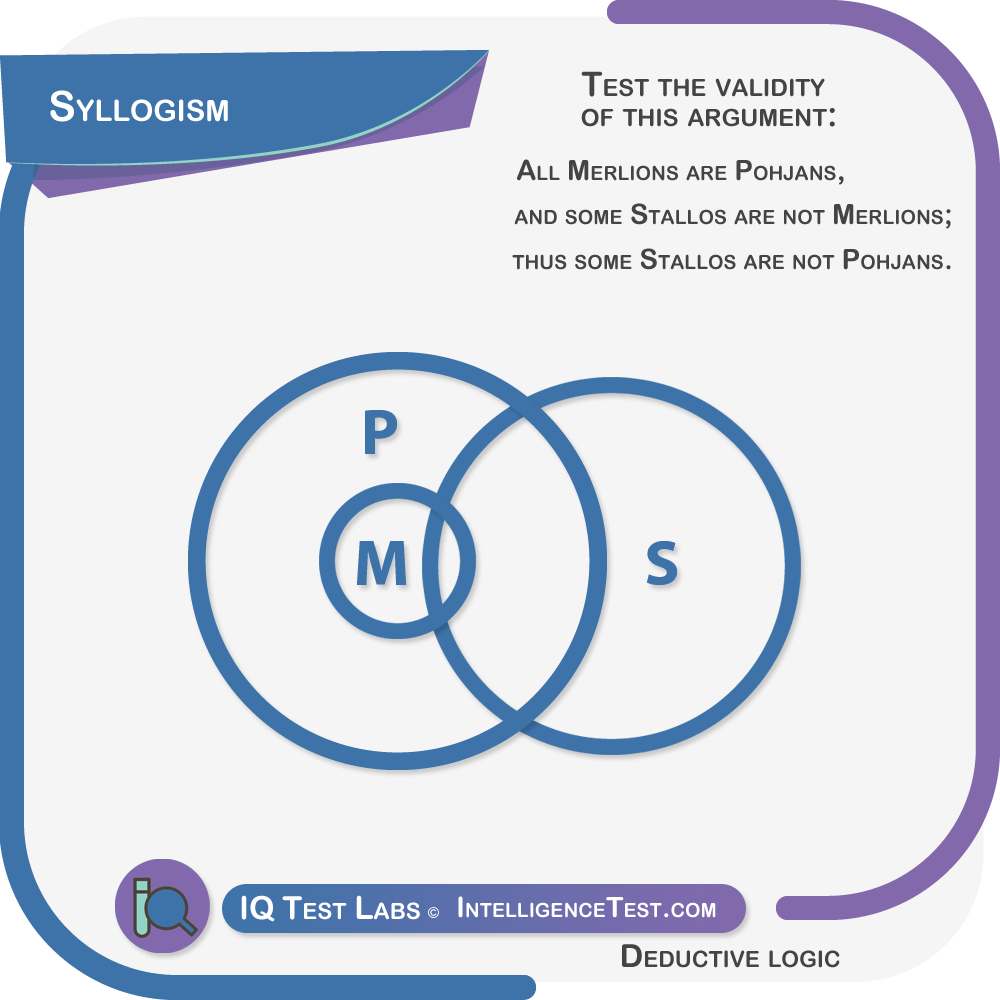
1. All Merlians are Pojhans.
2. Some Stallos are not Merlions.
Conclusion: Some Stallos are not Pohjans.
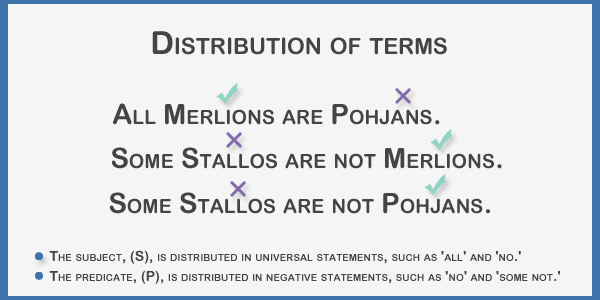
Conclusion is not valid
Rule 5: Whereas the major term, P, is distributed in the conclusion, it is not distributed in the major premise.
Example 8:
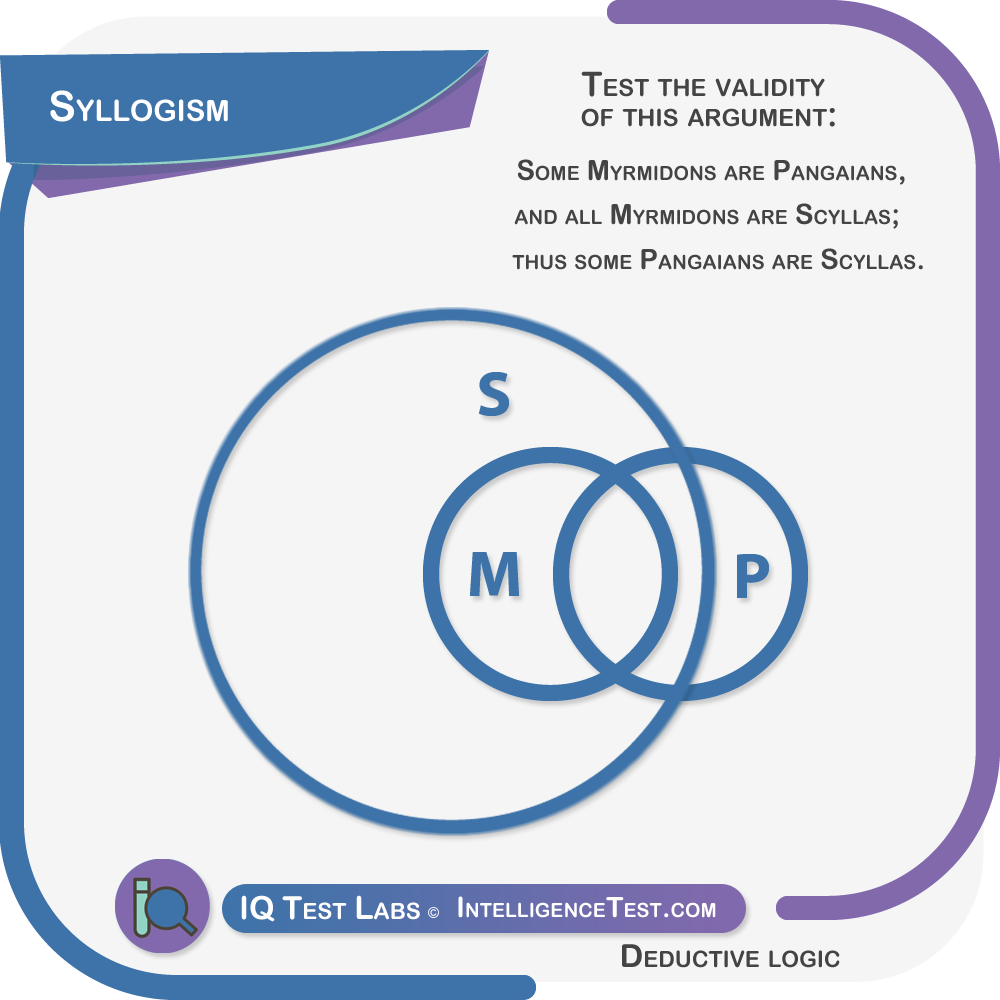
1. Some Myrmidons are Pangaians.
2. All Myrmidons are Scyllas.
Conclusion: Some Pangaians are Scyllas.
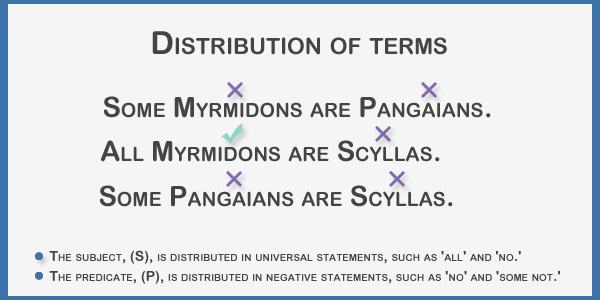
Conclusion is valid
Similar to case 7.
Rule 1: When both premises are positive, then the conclusion must be positive.
Rule 3: The middle term, B, is distributed in the minor premise.
Rule 9: If a statement begins with 'some', the conclusion must begin with 'some'.